Radical Impressionist: A Mathematician Paints

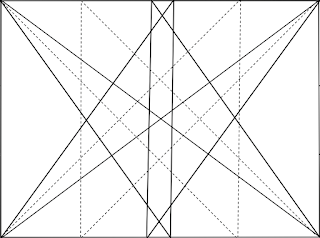
Expanded Dynamic Symmetry Armature We will construct an expanded dynamic symmetry armature to include the diagonals, their reciprocals and rebated squares.  These elements have been used by artists throughout history. We begin with main diagonals and one reciprocal.  We call the intersection of the diagonal with its reciprocal a polar point.  Recall that a reciprocal is perpendicular to a diagonal by definition or they meet at 90 degrees.  We drop a vertical line from the intersection of the reciprocal with the top edge to the bottom edge.  We shall prove that the vertical rectangle created with this dropped vertical as the left edge has the same aspect ratio as the main rectangle. First the triangles formed by the reciprocal and diagonal are marked with angle a and angle b as indicated. From Euclidean geometry we see that angle a plus angle b + 90 = 180 degrees. And angle a plus angle b = 90 degrees. Note the upper right corner is a 90 degree angle.  Therefore the two component angles

  Luminous Lands III with Expanded Dynamic Symmetry Armature This post illustrates:  the use of an expanded dynamic symmetry armature,  cropping of a photo to help compose a painting that would follow the armature,  drawing of the armature on the panel sketch on top of the armature  adjustments made throughout the painting process. In the case of this painting, I originally tried to do it on oil paper, but decided it didn't work for me.  the resulting intermediate painting became the source for the final painting on linen on panel.  painted a version with a golden proportion (or near) of 8x13. For that painting I used a golden spiral armature, though this is not illustrated here (yet). Photo of the cliffs in Los Alamos New Mexico: I cropped the photo so that the darker cliff against the lighter one would be set as my focus. I then used Wise Photo App to make sure it aligned as I expected: I used an expanded dynamic symmetry armature, with self-similar rectangles and rebatement to c

This is the story of an 8x13 painting that changed completely; note that 8 and 13 are two numbers in the Fibonacci sequence (1,1,2,3,5,8,13,21, etc.). Also its aspect ratio is close to the golden mean (13/8 = 1.625, while the golden mean is 1.618...)  The original intent was to capture the colors of a southwest landscape with distant mountains. The painting was moderately successful. I had done a series of "chromatic" paintings of the southwest landscape as well as aspen-covered mountains of New Mexico.  As I prepared for my Los Alamos show in July 2023, I wanted to change the painting to a more abstract painting emphasizing cliffs, using or imagining a dynamic symmetry armature and golden spiral grid. I used a squeegee for a new version as it is very useful in rendering straight edges. Sequence of original painting steps: Two years LATER (April 2023) I decided to change this 8x13 painting.  After sanding... Half an hour later using a squeegee to add strokes: Land Abstraction

The 2023 Los Alamos Paint Out, sponsored by the Plein Air Painters of New Mexico,  took place July 28 - Aug 2.   After the six days of painting we were to choose two paintings to be displayed at the Fuller Lodge Art Center Gallery for the month of August. Visit  https://www.papnm.org/2023-Los-Alamos-Paint-Out-and-Show/ .  The gallery is in the center of town, a historic building that had once housed a school in Los Alamos. See  Oppenheimer had been a student there and in fact liked the area so much that he chose it for the Manhattan Project during World War II at the Los Alamos Laboratory (current name; of course during the War this was all very secret). On the first paint out day, we headed over to the White Rock Overlook 20 minutes away to paint a scene I've painted many times. There's an alternate view with the Rio Grande down a canyon that I will paint someday. But I would need to trek a little.  I chose instead to paint in the other direction toward the Black Mesa (in the